Appendix E: A Detailed Look at f 253
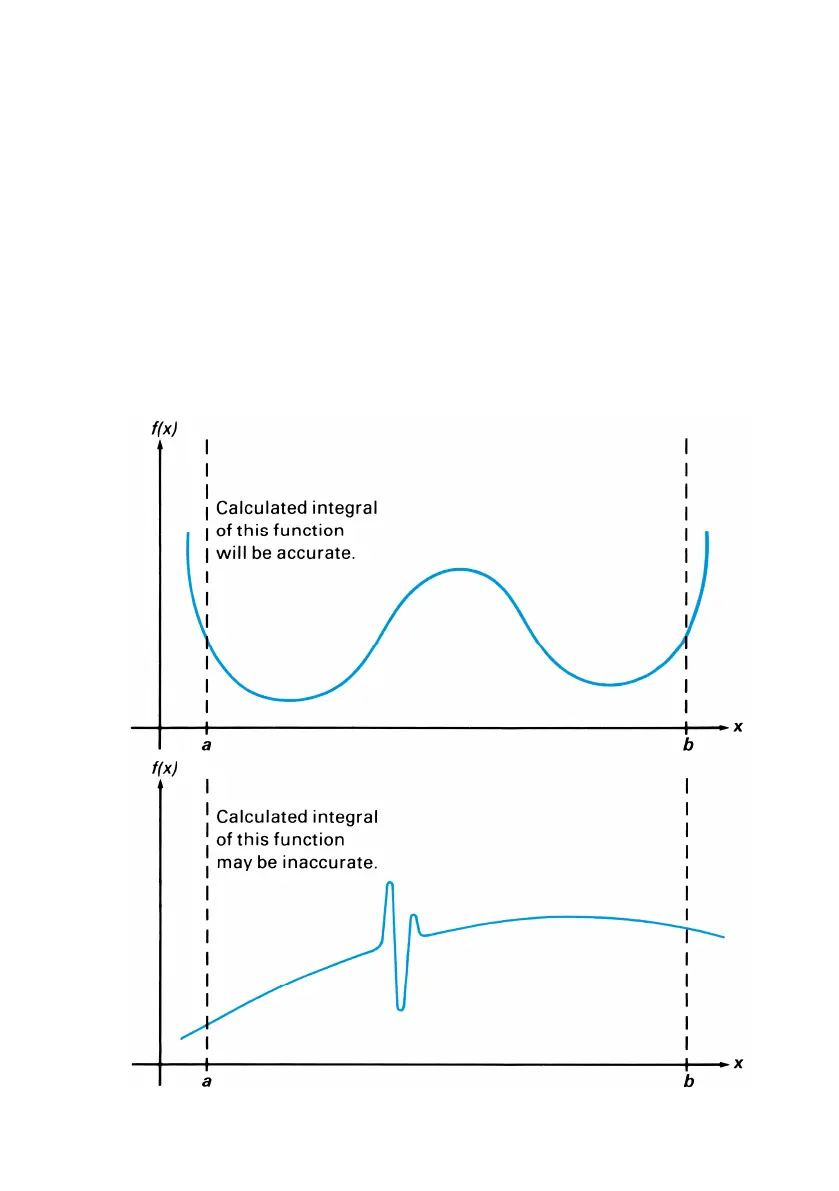
Note that the rapidity of variation in the function (or its low-order
derivatives) must be determined with respect to the width of the interval
of integration. With a given number of sample points, a function f (x) that
has three fluctuations can be better characterized by its samples when
these variations are spread out over most of the interval of integration
than if they are confined to only a small fraction of the interval. (These
two situations are shown in the next two illustrations.) Considering the
variations or fluctuations as a type of oscillation in the function, the
criterion of interest is the ratio of the period of the oscillations to the
width of the interval of integration: the larger this ratio, the more quickly
the algorithm will terminate, and the more reliable will be the resulting
approximation.
To Next Page
Loading...
Need help?
General
