10 Rockwell Automation Publication 750-AT006D-EN-P - January 2022
Chapter 1 Background
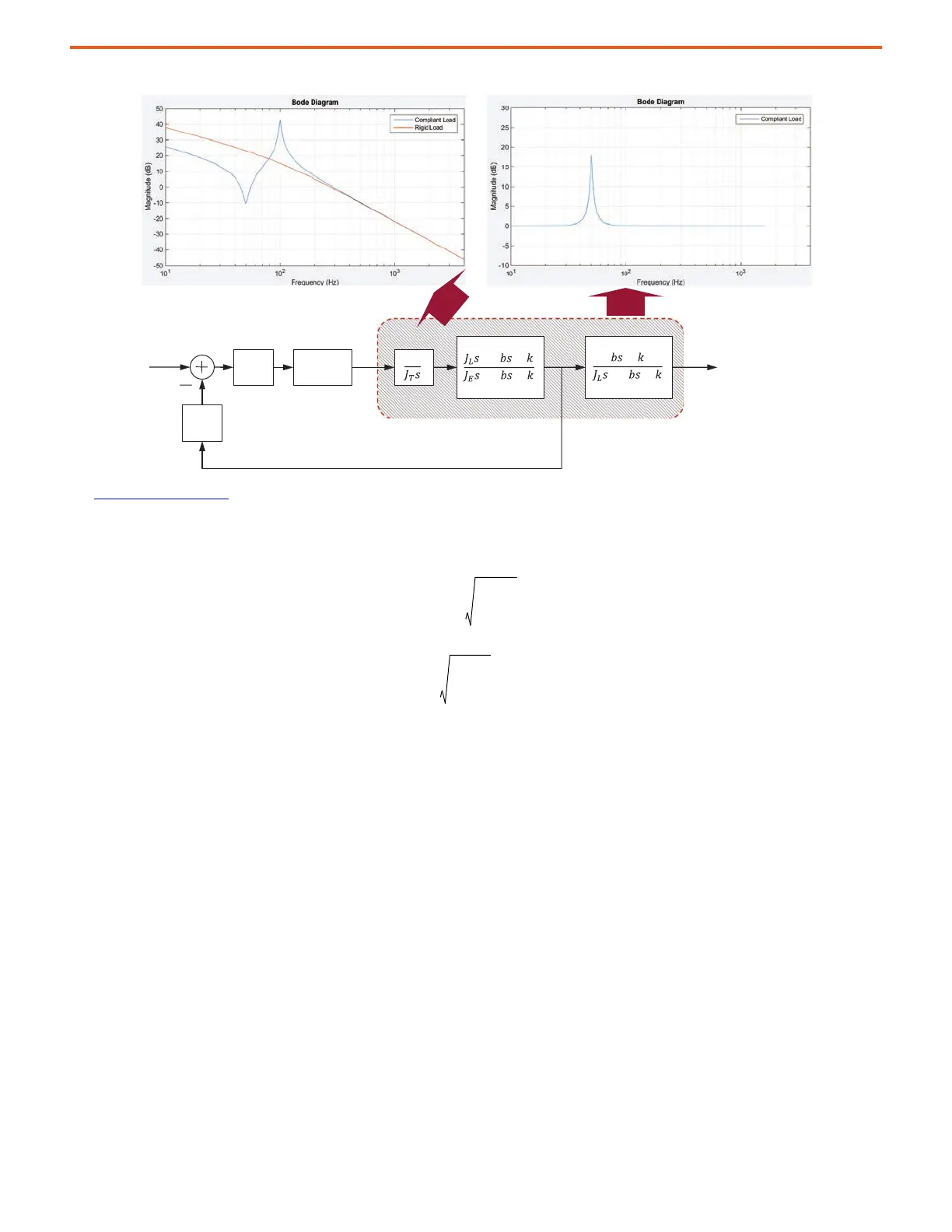
Figure 4 - Compliant Load Resonances
See Resonances
on page 12 for information on the types of resonances, how they affect tuning, and how to control them.
The resonant frequency F
R
and anti-resonant frequency F
A
can be calculated in units of [Hz], with an equivalent inertia J
E
that is used in the
following calculations.
The severity of this tuning problem increases under the following conditions:
• As the spring constant decreases, the coupling becomes less rigid and twists more.
• As the load ratio increases, the load on the end of the spring becomes larger, which causes it to twist more. Larger load ratios amplify
tuning problems.
Compliance is also referred to as non-rigid. There are only two absolutes:
When k = 0, the load is disconnected
When k = ∞, the load is rigid
When 0 < k < ∞, everything else is compliant and/or has backlash. This condition constitutes most industrial applications over a wide range
of dynamic behaviors.
Motor Side
Resonances
Load Side
Resonances
V
M
V
CMD
1
Power
Converter
T
m
PI
LPF
2
+ +
2
+ +
+
2
+ +
V
L
Velocity Loop
F
R
k
J
E
4
2
--------------- -
=
F
A
k
J
L
4
2
---------------
F
R
R 1+
------------ -==
J
E
J
L
J
M
J
L
J
M
+
--------------------
J
M
R
R 1+
------------ -
==
To Next Page
Loading...
Need help?
General
