Harmonics algorithm. Harmonics are the sinusoidal voltages or currents having frequencies that are integer multiples of the
frequency at which the supply system is designed to operate (termed the fundamental frequency). Distorted waveforms can be
decomposed into sum of the fundamental frequency and its harmonics.
■
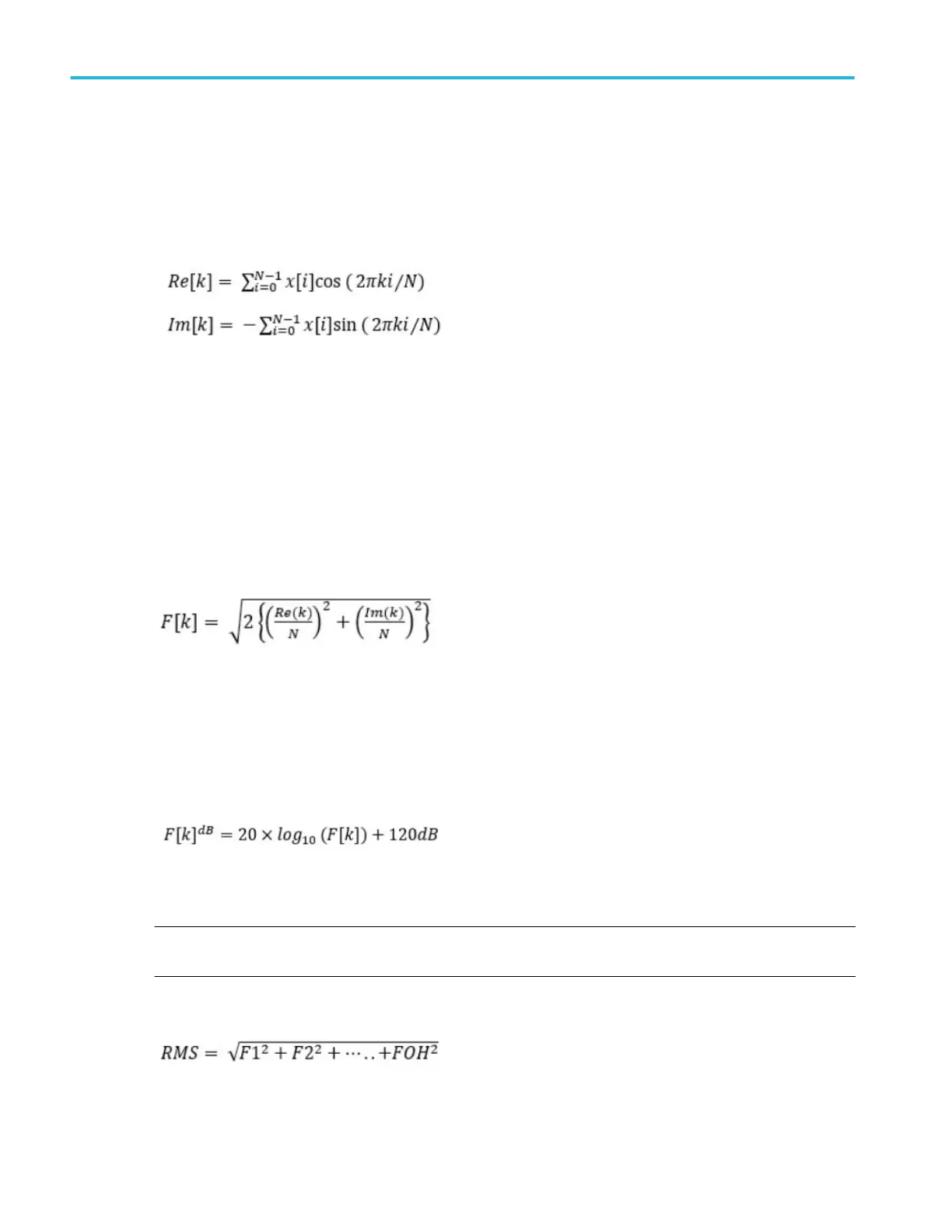
The measurement uses the Discrete Fourier Transform (DFT) to calculate the Real component (Re(k)) and Imaginary
component Im(k). The Real component (Re(k)) and Imaginary component Im(k) are calculated using the following equation:
Where:
Re[k] is the Real component of kth harmonic.
Im[k] is the Imaginary component of kth harmonic.
i is the Index of the input data value.
k is the Index of the harmonics, k index is calculated using the harmonics number.
x[i] is the discrete set of acquire time samples.
■
Harmonics Fk is calculated using the following equation:
Where:
F[k] is the kth harmonic, in Amp/Volt.
Unit of F[k] for voltage signal is Volt and for current signal is Ampere.
Harmonics is converted into dB using the following equation:
F[k]
dB
is the k
th
harmonic, dBA or dBV based on the harmonics input source configuration.
NOTE. In case of 'AM 14' standard, acquired signal is divided into 15 chunks and all harmonics are calculated for each
chunk. For each individual harmonics maximum is taken out of 15 values.
■
RMS: The RMS of harmonics is calculated in unit of volt or ampere using the following equation:
F1 is the fundamental harmonics or line harmonics.
Measurement algorithms
464 MSO54, MSO56, MSO58, MSO58LP, MSO64 Help

 Loading...
Loading...