© 2019 Thorlabs GmbH
8 Tutorial
58
8.1.3 Mathematical Representation of an Electromagnetic Wave
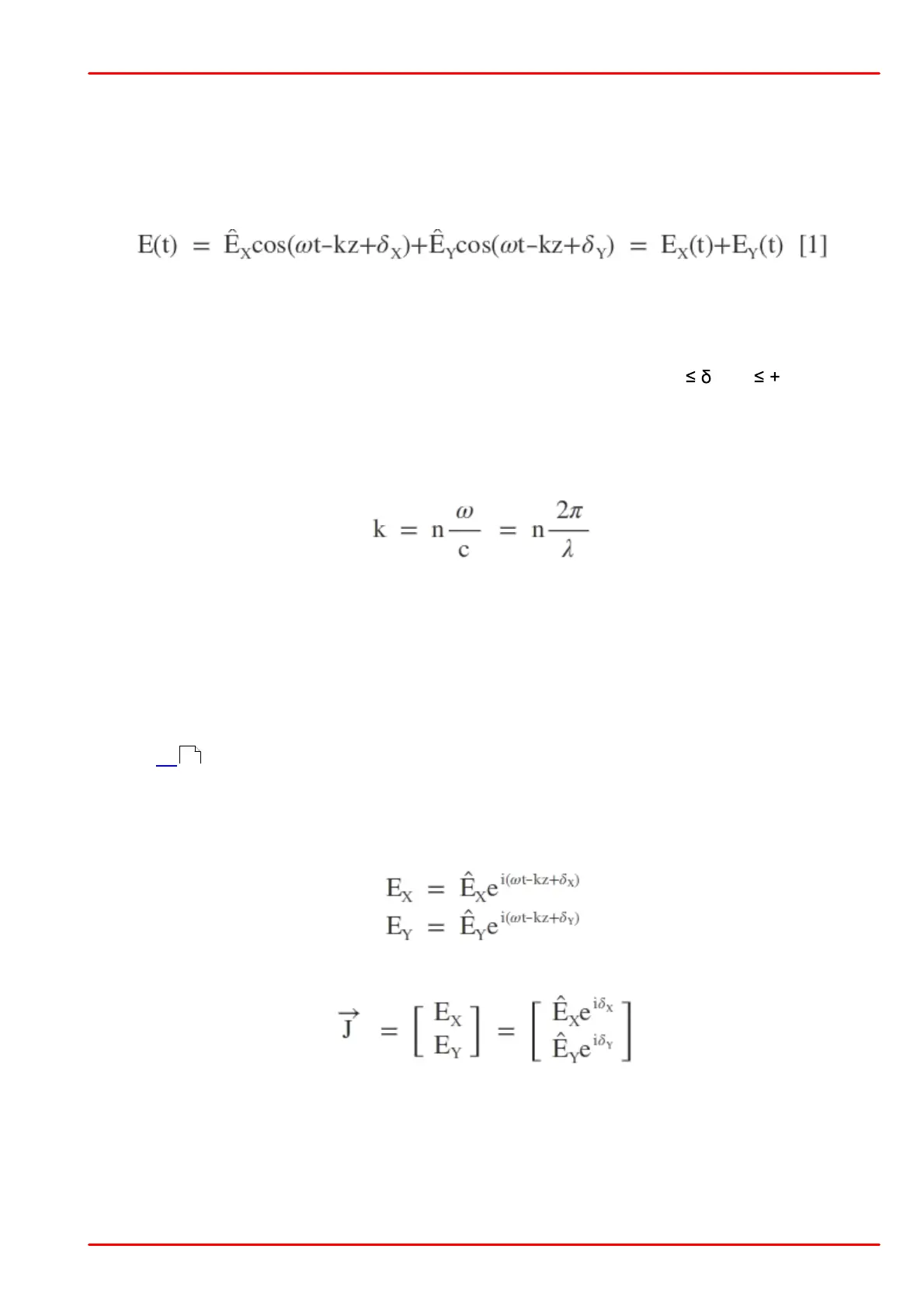
An electromagnetic wave of frequency w traveling in Z direction has its polarization vector in the
X-Y plane. The E-field direction is always perpendicular to the direction of propagation. Any loc-
ation of the polarization vector at different times t can be written by superposition of the X- and
Y- vector components:
with:
Ê
X
, Ê
Y
Amplitudes of the electric field intensity in X or Y direction
E
X
, E
Y
Instantaneous electrical field intensity in X or Y direction
δ
X
, δ
Y
Phase of the electric field intensity in X or Y direction, -p
X
, δ
Y
p
w Angular frequency
k Wavenumber
The wavenumber k can be calculated by the frequency or wavelength:
with:
n Refraction index
c Speed of light
l Wavelength in vacuum
Jones Vector Formalism
In optics, polarized light can be described using the Jones calculus, developed by R. C. Jones
in 1941 [1] . Polarized light is represented by a Jones vector, and linear optical elements are
represented by Jones matrices. The Jones vector describes the polarization of light in free
space or another homogeneous isotropic non-attenuating medium, where the light can be prop-
erly described as transverse waves. Omitting the time dependency e
j
w
t
, the electromagnetic
wave can be fully characterized by the complex envelopes:
of the X and Y components of the electric field. The Jones vector is then
The Jones vector is not a vector in real space, but a mathematical abstraction in complex
space. The following figure shows the spatial curves of the X and Y components of the electric
field intensities as a function of time:
87
To Next Page
Loading...
Need help?
General
