© 2019 Thorlabs GmbH
8 Tutorial
60
8.1.4 Polarization Ellipse
The state of polarization can be represented by an ellipse:
The (virtual) spectator looks towards the light source.
Definition of right and left handed polarization:
The right hand rule is used to describe the handedness of the polarization state. The right hand
rule is used in a Cartesian coordinate system with X, Y, and Z axes, and the name of the rule
comes from the ability to use the right hand to apply the rule. The handedness of the polariza-
tion state can be determined from propagation direction, or vice versa. When determining the
handedness of the polarization state, point the thumb of the right hand along the direction of
light propagation. The fingers curl in the direction of the rotation of right hand polarized light
around the ellipse. Left hand polarized light propagates in the opposite direction.
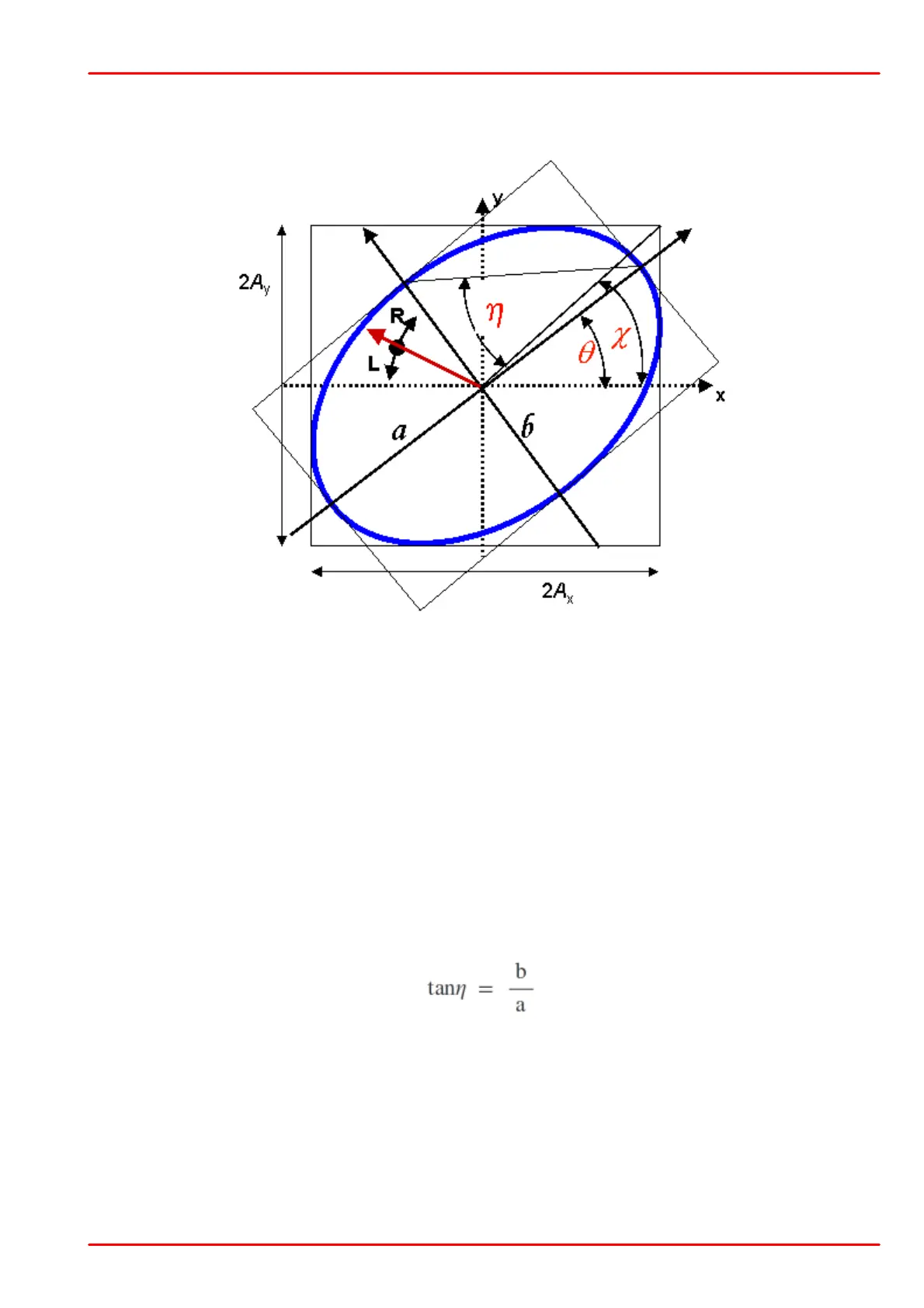
The parameters ellipticity (h) and azimuth (q) as well as the handedness (right and left which
corresponds to the sign of h) are necessary to uniquely describe the polarization. The azimuth
angle q is the angular deviation of the major axis of the ellipse from the X axis. The angle h is
calculated from the ratio of the semi-minor (b) to the semi-major (a) axis dimensions according
to the following equation:
h > 0: The light is right-handed polarized.
h = 0: The light is linear polarized.
h < 0: The light is left-handed polarized.
An ellipse can also be described by the parameters angle c and phase j. The handedness is
defined by the sign of j.
To Next Page
Loading...
Need help?
General
