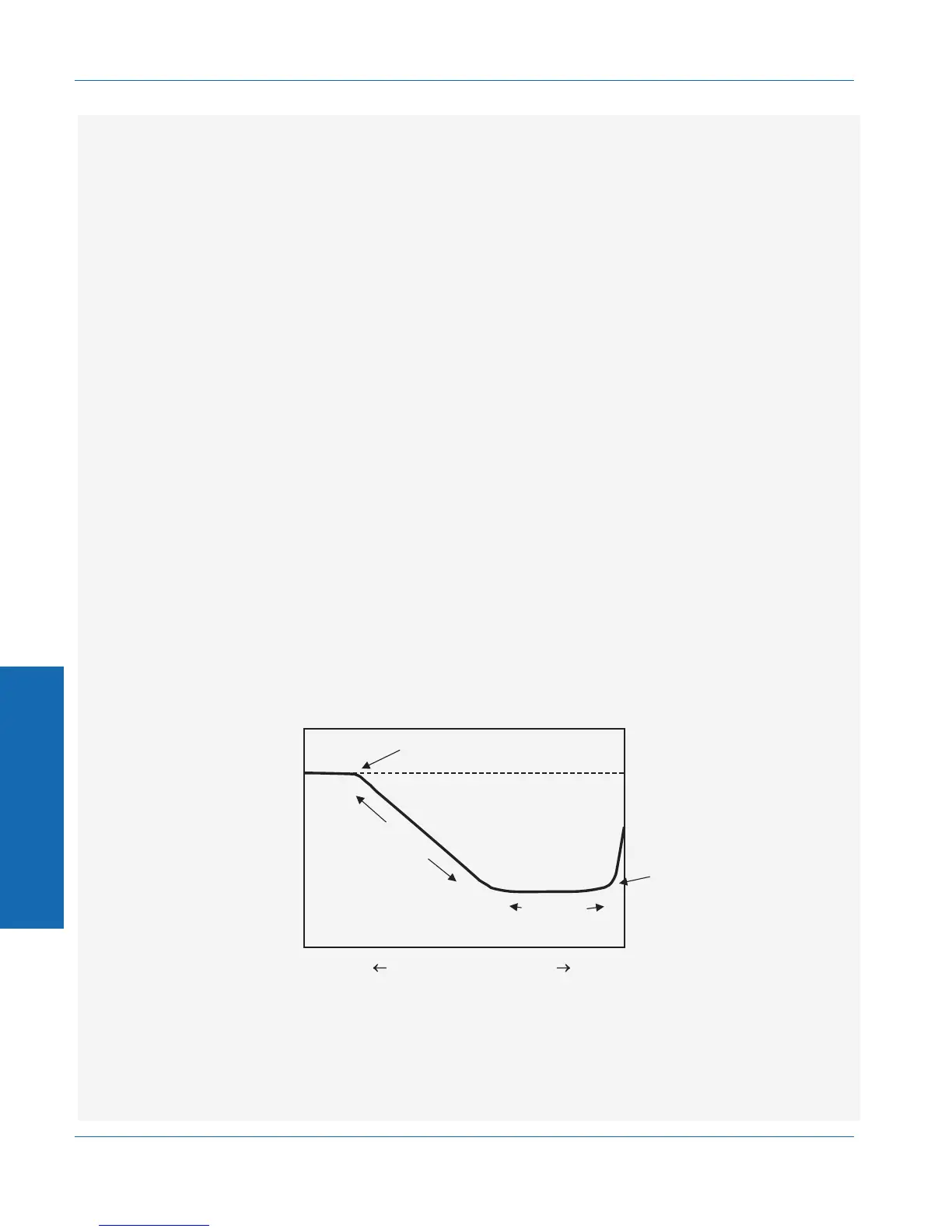
Changes with Amplitude
It is fairly common for the THD+N reading to apparently increase as the fundamental
tone amplitude decreases, even if the device does not create more distortion or noise at
lower amplitudes.
This effect is due to the fact that the THD+N reading is the ratio of the amplitude before
filtering to the amplitude after filtering. If the amplitude after filtering is dominated by
noise, hum, or interference, it will be essentially constant regardless of the amplitude of
the fundamental tone. Therefore, as the amplitude of the fundamental tone decreases
there will be an apparent increase in the calculated ratio.
Since all devices create some noise, any device has a ‘noise floor’. This is the level of
noise that is always created by the device. If the fundamental tone has a lower
amplitude than the noise floor, there will be essentially no difference between the level
of filtered and unfiltered signals and the THD+N will be 100%.
In some devices, the unwanted signals may actually increase as signal amplitude
decreases. If you suspect this, you may want to make an absolute THD+N
measurement, which does not include the amplitude before filtering in the
measurement. This is accomplished by selecting any unit besides % or dB.
All devices also have a maximum allowable amplitude. If the fundamental tone is above
this amplitude, the device will generally ‘clip’, creating very high distortion. Almost any
device will show very high distortion readings at maximum or near-maximum amplitude.
The following graph shows common THD+N characteristics as a function of amplitude:
100% (0 dB)
THD+N
(Log Scale)
Amplitude
(Log Scale)
higher
lower
Fundamental has
reached noise floor
THD+N is
dominated
by noise
THD+N is
dominated
by distortion
Clipping
threshold
Figure 4-18. THD + N characteristics as a function of amplitude
4 Functions
THD+N (Total Harmonic Distortion plus Noise) Function Descriptions
4-18 ATS-1 Dual Domain User's Manual

 Loading...
Loading...