55
3-2 Differential Calculations [OPTN]-[CALC]-[d/dx]
To perform differential calculations, first display the function analysis menu, and
then input the values shown in the formula below.
2(d/dx) f(x),a,A x)
The differentiation for this type of calculation is defined as:
In this definition,
infinitesimal
is replaced by a
sufficiently small
Ax, with the value
in the neighborhood of f ' (a) calculated as:
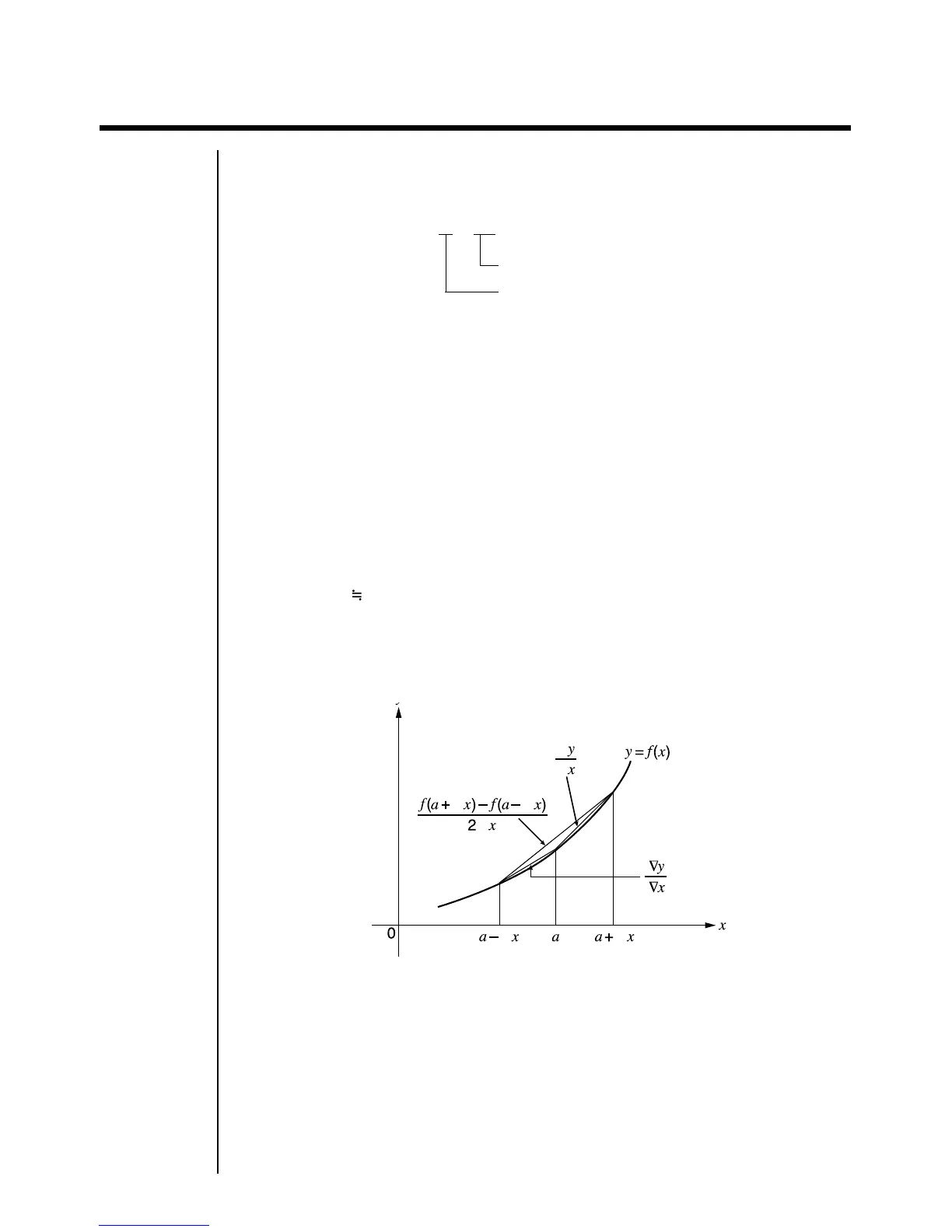
In order to provide the best precision possible, this unit employs central difference
to perform differential calculations. The following illustrates central difference.
A
A
AA
A
AA
The slopes of point a and point a + Ax, and of point a and point a – Ax in function
y = f(x) are as follows:
In the above, Ay/Ax is called the forward difference, while ∇y/∇x is the backward
difference. To calculate derivatives, the unit takes the average between the value
of Ay/Ax and ∇y/∇x, thereby providing higher precision for derivatives.
f (a + Ax) – f (a)
f '(a) = lim –––––––––––––
Ax
Ax→0
f (a + Ax) – f (a) Ay f (a) – f (a – Ax) ∇y
––––––––––––– = ––– , ––––––––––––– = –––
Ax Ax Ax ∇x
f (a + Ax) – f (a)
f '(a) –––––––––––––
Ax
Increase/decrease of
x
Point for which you want to determine the derivative
d
d/dx ( f (x), a, Ax) ⇒ ––– f (a)
dx
To Next Page
Loading...
Need help?
General
