Displacement power
factor (cosφ/ DPF)
0…1
50% U
nom
≤ U
RMS
< 150% U
nom
10% I
nom
≤ I
RMS
< I
nom
(1) See section 10.2.9 Estimated measurement uncertainty values for power and energy
10.2.9 Estimated measurement uncertainty values for power and energy
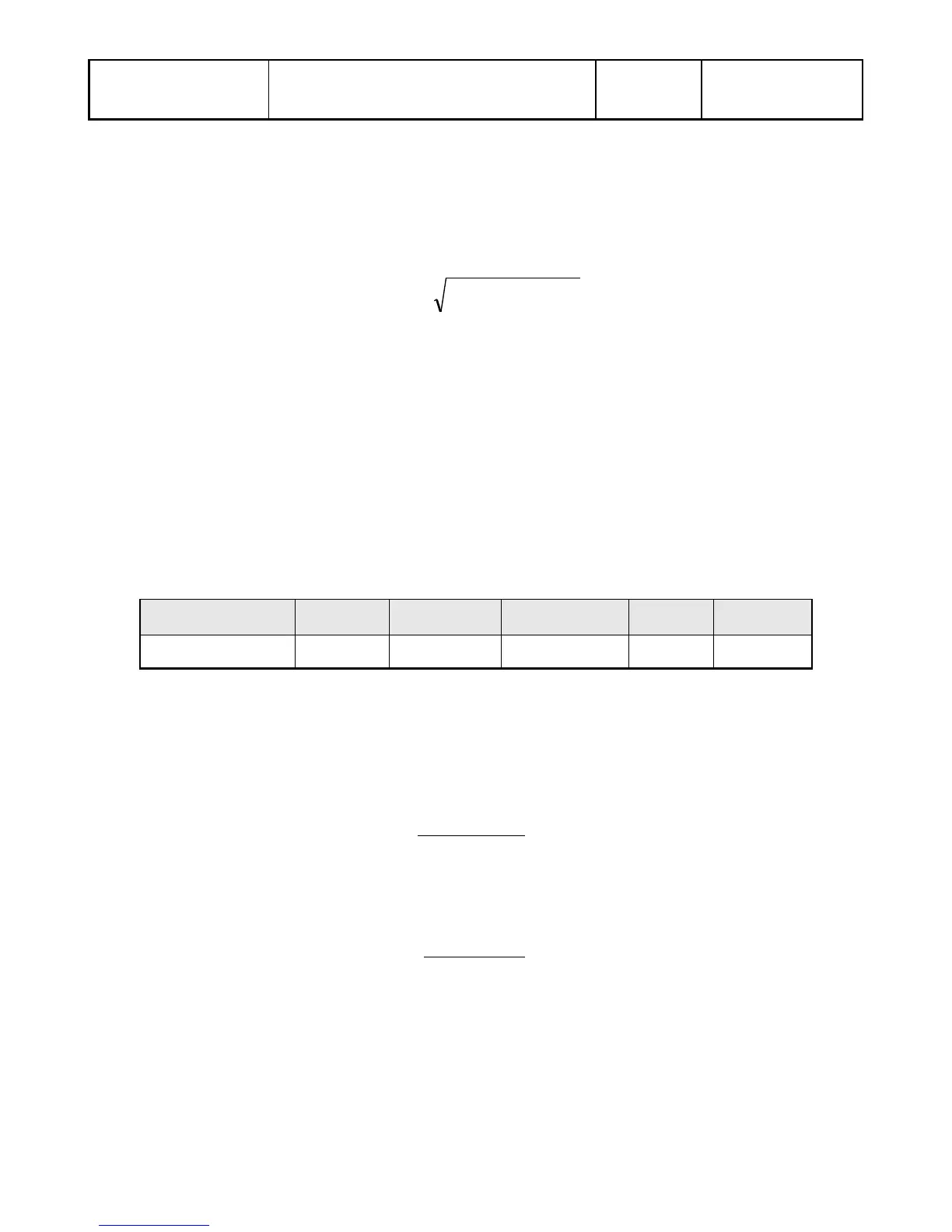
The total measurement uncertainty for power, active and reactive energy (fundamental compo-
nent) is based on the following relation (for energy we ignore the additional uncertainty due to time
measurement, as it is much smaller than other uncertainties):
where:
P,Q
– measurement uncertainty for active or reactive power,
Uh
– total measurement uncertainty of voltage harmonic amplitude (recorder, transduc-
ers,clamps),
Ih
– total measurement uncertainty of current harmonic amplitude (recorder, transducers,
clamps),
ph
– additional uncertainty of error in the measurement of the phase between voltage and
current harmonics.
The
ph
uncertainty may be calculated when the phase angle is known for the considered fre-
quency ban. Tab. 10.1 describes error of the phase difference between the voltage and harmonics for
MPI-540 recorder (without clamps and transducers).
Tab. 10.1. Phase error of PQM-540 recorder, depending on the frequency.
Phase error introduced by transducers and clamps may be usually found in their technical docu-
mentation. In this case, we need to estimate the resultant phase error between the voltage and the
current for a given frequency caused by all elements of the measuring circuit: current and voltage
transducers, clamps, and the recorder.
The uncertainty of the specific harmonics active power measurements may be calculated according to
the following formula:
0cos [%],
cos
cos
1100
ph
On the other hand, the uncertainty of the harmonics reactive power measurement may be calculated
according to the following formula:
0sin [%],
sin
sin
1100
ph
In both formulas, means the actual phase shift angle between the current and voltage components,
and means the total phase error for a given frequency.

 Loading...
Loading...