Oscilloscope Reference Offset, position, scale, and math waveforms
Offset, position, scale, and math waveforms
The settings that you make for offset, scale, and position affect the math waveform you obtain. Here are
some tips for obtaining a good display:
Scale and position the source waveform so that it is contained on the screen. (Off-screen waveforms
may be clipped, resulting in errors in the derivative waveform).
Use vertical position a nd vertical offset to position your source waveform. The vertical position
and offset will not affect your derivative waveform unless you position the source waveform off
screen so that it is clipped.
What do you want to do next?
Learn about w aveform integration. (see page 750)
Go to a step-by-step procedure for creating math waveforms. (see page 612)
Go to a step-by step procedure for using math waveforms. (see page 606)
Waveform integration
The math capabilities of the instrument include waveform integration. This allows you to display an
integral math waveform that is an integrated version of the acquired waveform.
Use integral waveforms in the following applications:
Measuring power and energy, such as in switching power supplies.
Characterizing mechanical transducers, as when integrating the output of an accelerometer to obtain
velocity.
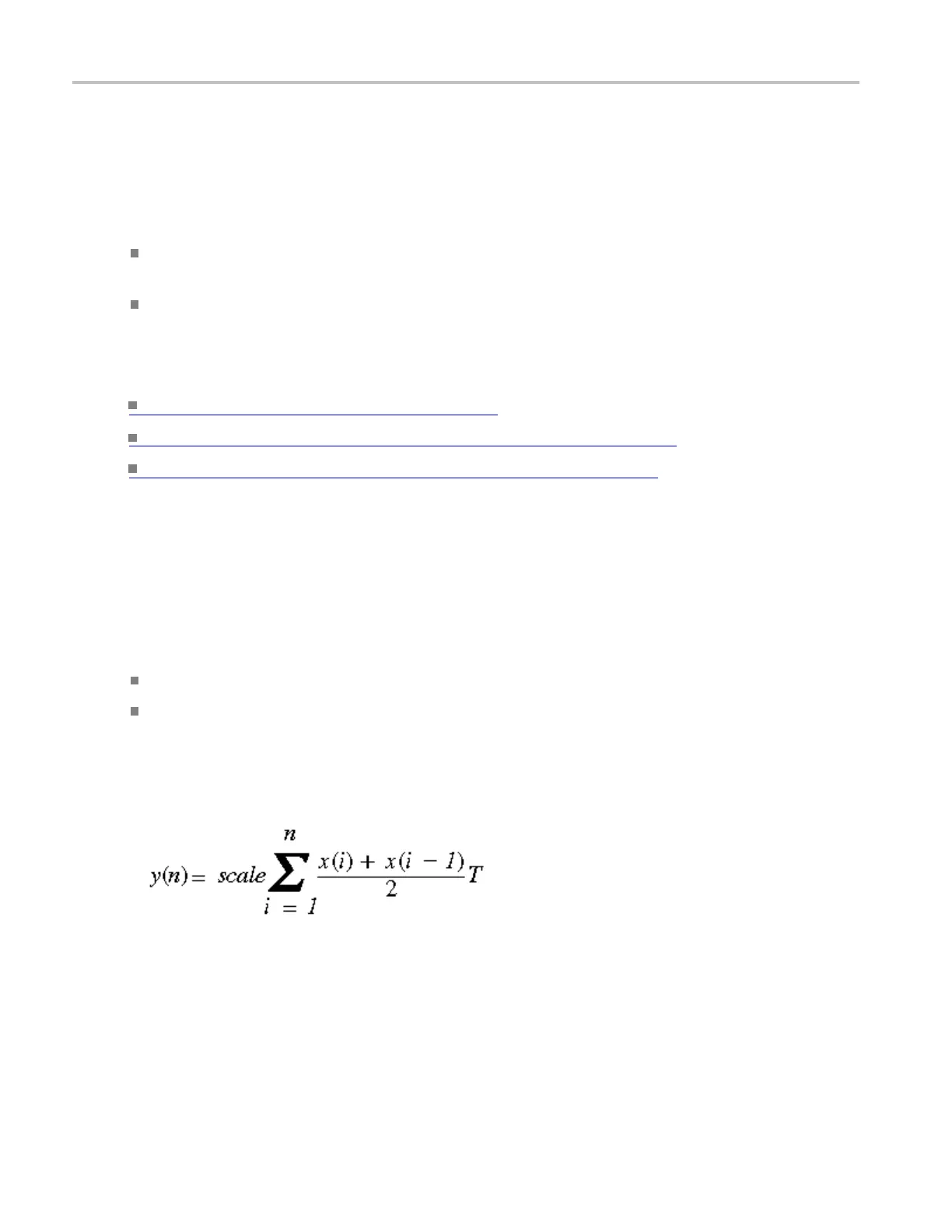
The integral math waveform, derived from the s ampled waveform, is computed based on the following
equation:
Where: x(i) is the source waveform, y(n) is a point in the integral math waveform, scale is the output
scale factor, and T is the time between samples.
Since the resultant math waveform is an integral waveform, its vertical scale is in volt-seconds (its
horizontal scale is in seconds). The source signal is integrated over its entire record length; therefore, the
math waveform record length equals that of the source waveform.
750 DSA/DPO70000D, MSO/DPO/DSA70000C, DPO7000C, and MSO/DPO5000 Series
To Next Page
Loading...
Need help?
General
