Chapter 10
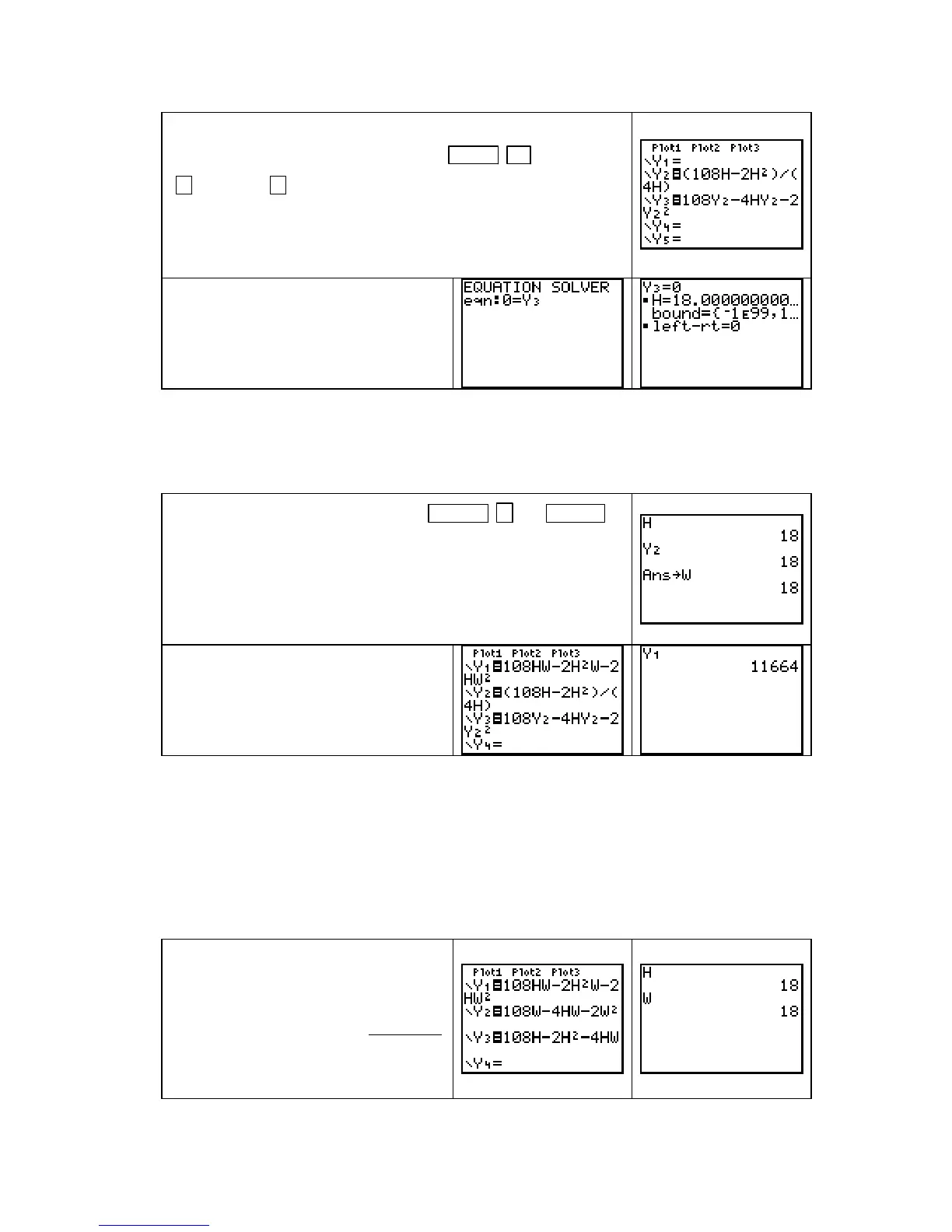
Now, replace every
W in Y3 with the symbol Y2 by placing the
cursor on each
W location and pressing VARS ► [Y−VARS]
1
[Function] 2 [Y2]. What you have just done is substitute
the expression for
W from equation 2 in equation 1.
The expression in
Y3 is the left-hand side of an equation that
equals 0 and it contains only one variable, namely h.
The next step is to use the SOLVER to
solve the equation
Y3 = 0. Try differ-
ent guesses and see that they all result
in the same solution for this particular
equation.
WARNING: You need to closely examine the equation in Y3 and see what type of function
it represents. In this case,
Y3 contains H to no power higher than one, so it is a linear equation
and has only one root. If
Y3 contains the variable squared, the equation is quadratic and you
need to try different guesses because there could be two solutions, and so forth.
Return to the home screen and press ALPHA ^ [H] ENTER
to check that the calculator knows that the answer from the
SOLVER is a value of H.
Next, find w by evaluating
Y2. The calculator does not know
that the output of
Y2 equals W, so be sure to store this value in
W.
The last thing to do is to find the out-
put V(h, w) at the current values of h
and w. To do this, enter the function
V in
Y1 and call up Y1 on the home
screen.
The critical point has coordinates h = 18 inches, w = 18 inches, and V = 11,664 cubic inches.
CLASSIFYING CRITICAL POINTS USING THE DETERMINANT TEST Once you
find one or more critical points, the next step is to classify each as a point at which a maximum,
a minimum, or a saddle point occurs. The Determinant Test often will give the answer. Also,
because this test uses derivatives, the calculator’s numerical derivative
nDeriv can help.
We illustrate with the critical point that was found in the previous example of this Guide.
To use the Determinant Test, we need to calculate the four second partial derivatives of V and
then evaluate them at the critical point values of h and w.
Enter the functions V in
Y1, V
h
in Y2
and V
w
in
Y3. These quantities are
given on page 105 of this Guide.
H and W should contain the unrounded
values of the inputs at the critical point.
(Here,
H and W are integers, but this
will not always be the case.)
Copyright © Houghton Mifflin Company. All rights reserved.
106

 Loading...
Loading...

















