Chapter 7
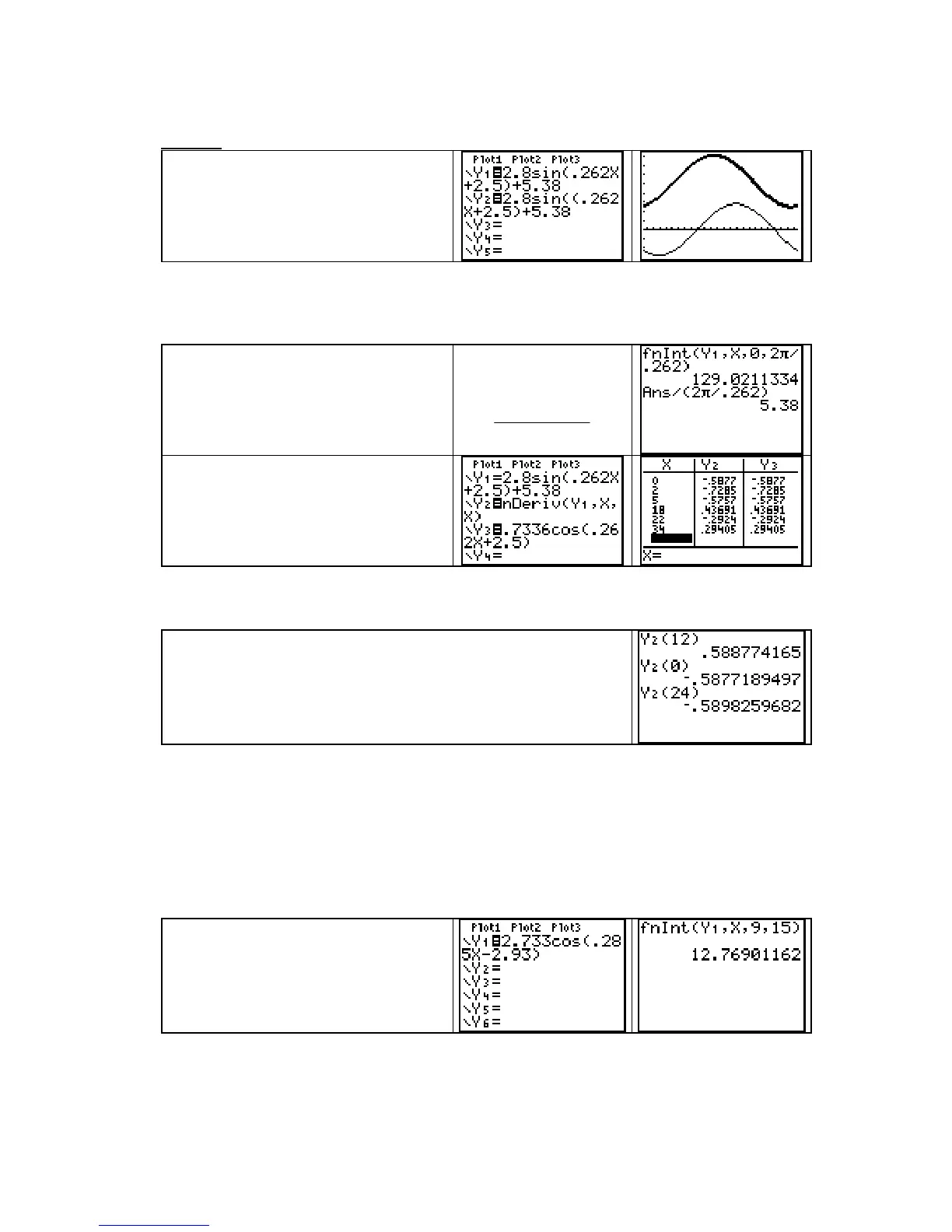
and not have the correct equation entered. The function (see below) that is entered in
Y2 is
incorrect.
The correct way to enter the function c
is shown in
Y1. When these two equa-
tions are graphed, you can see that they
are entirely different functions. The
correct function is the darker one.
Delete the incorrect function in Y2. We now return to Example 1. Part a asks for the average
number of calls the county sheriff’s department receives each hour. The easiest way to obtain
this answer is to remember that the parameter k in the sine function is the average value.
You can also find the average value
over one period of the function using
the methods on page 80 of this guide.
Enter the function c in
Y1 and type in
the quotient shown to the right.
Average Value =
( ) ct dt
0
2 0 262
202620
π
/.
/.
−
Enter the calculator’s numerical
derivative in
Y2. Enter your answer to
part b, the formula for c′(t), in
Y3You
may use the
TABLE or the graphs of Y2
and
Y3 to check your answer.
Part c of Example 1 asks how quickly the number of calls received each hour is changing at
noon and at midnight.
To answer these questions, simply evaluate
Y2 (or your deriva-
tive in
Y3) at 12 for noon and 0 (or 24) for midnight. (You were
not told if “midnight” refers to the initial time or 24 hours after
that initial time.)
7.5 Accumulation in Cycles
As with the other functions we have studied, applications of accumulated change with the sine
and cosine functions involve the calculator’s numerical integrator
fnInt.
INTEGRALS OF SINE AND COSINE FUNCTIONS
We illustrate the process of
determining accumulated change with Example 1 in Section 7.5 of Calculus Concepts.
Enter the rate of change of tempera-
ture in Philadelphia on August 27,
1993 in
Y1. Find the accumulated
change in the temperature between
9 a.m. and 3 p.m. using
fnInt.
The temperature increased by about 13
o
F between 9 a.m. and 3 p.m.
Copyright © Houghton Mifflin Company. All rights reserved.
92
To Next Page
Loading...
Need help?
General
