TI-83, TI-83 Plus, TI-84 Plus Guide
regression in the calculator, it is sometimes necessary to have an estimate of the period of the
data.
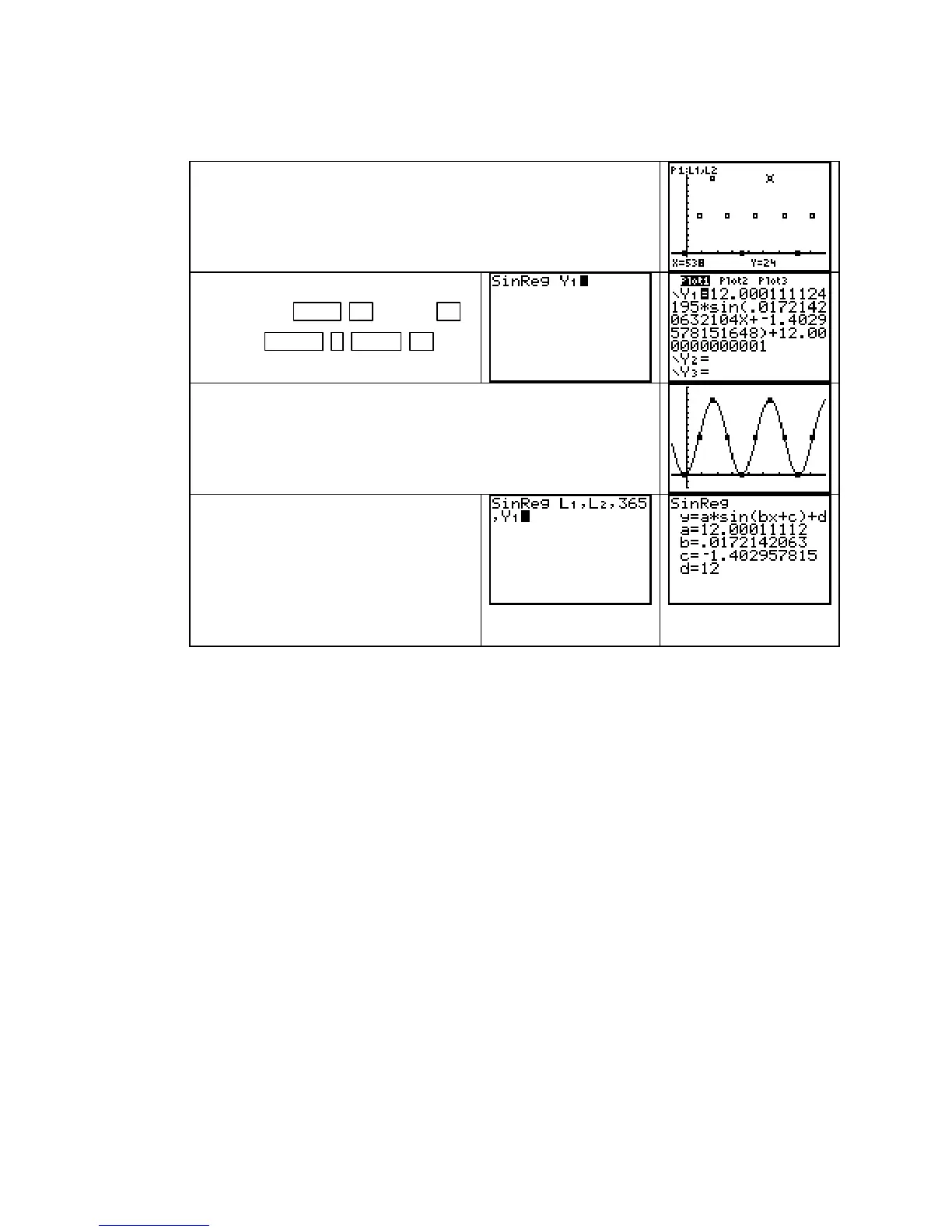
The data appear to be cyclic. Either view the data or TRACE
the scatter plot to measure the horizontal distance between one
high point and the next (or between any two successive low
points). One cycle of the data appears to be about 538 – 173 =
365 days.
Fit a sine function to the data and paste
it in
Y1 with STAT ► [CALC] ▲
[SinReg]
ENTER , VARS ►
[Y−VARS] 1 [Function] 1 [Y1].
Graph the model on the scatter plot of the data.
(If the graph of the function looks like a line, you have not set
the
MODE menu to Radians!)
Even though it did not occur in this
example, you may get a
SINGULAR
MATRIX
error when trying to fit a
sine model to certain data. If so, try
specifying an estimate for the period
of the model. You also need to specify
the data location with the period.
Recall that our estimate
of the period is 365 days.
For these data, the same
function results.
NOTE: If you do not think the original function the calculator finds fits the data very well,
try specifying a period and see if a better-fitting equation results. It didn’t here, but it might
with a different set of data. If you still cannot find an equation, you can tell the calculator
how many times to go through the routine that finds the equation. This number of iterations
is 3 if not specified. The number should be typed before
L1 when initially finding the
equation.
7.3 Rates of Change and Derivatives
All the previous techniques given for other functions also hold for the sine model. You can
find intersections, maxima, minima, inflection points, derivatives, integrals, and so forth.
DERIVATIVES OF SINE AND COSINE FUNCTIONS Evaluate
nDeriv at a particular
input to find the value of the derivative of the sine function at that input. We illustrate with
Example 1 in Section 7.3 of Calculus Concepts:
The calls for service made to a county sheriff’s department in a certain rural/subur-
ban county can be modeled as c(t) = 2.8 sin(0.262t + 2.5) + 5.38 calls during the tth
hour after midnight.
CAUTION: Because the calculator automatically inserts a left parenthesis when you press
the sine
and cosine keys, be very careful that you do not type an extra parenthesis when
entering a trig function in the graphing list. If you do, you will change the order of operations
Copyright © Houghton Mifflin Company. All rights reserved.
91

 Loading...
Loading...

















