Chapter 2
next is type
Y2 and press ENTER . Then, store the next set of inputs into A and/or B and
recall
Y2, using 2nd ENTER (ENTRY) to recall each instruction. Press ENTER and you
have
the average rate of change between the two new points. Try it.
FINDING PERCENTAGE CHANGE You can find percentage changes using data either
by the formula or by using program
DIFF. To find a percentage change from a function
instead of data, you should use the percentage change formula. We again use the function
describing the temperature on a typical day in May in a certain Midwestern city that is given in
Example 3 of Section 2.1 of Calculus Concepts to illustrate.
Have T(t) = in Y1. The percentage change in
the temperature on a typical day in May in a certain Midwestern
city between 11:30
A.M. and 6 P.M. is given by the formula
2
0.8 2 79 F−++tt
o
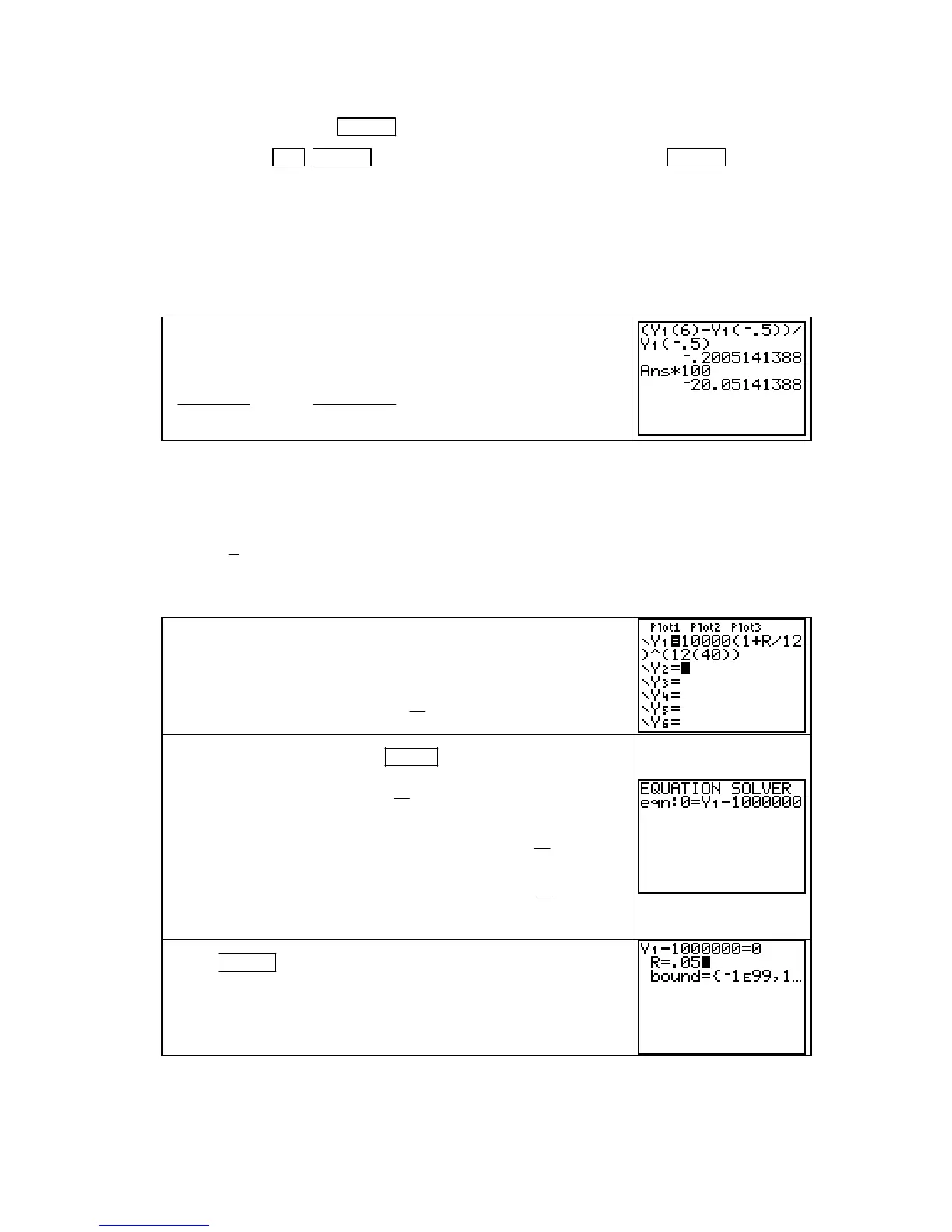
(6) ( 0.5)
(0.5)
100%
−−
−
⋅
TT
T
=
Y1(6) Y1( 0.5)
Y1( 0.5)
100%
−−
−
⋅ . Recall that if you type in
the entire quotient, you avoid rounding errors.
The temperature on a typical day in May in a certain Midwestern city decreased by about
20.05% between 11:30
A.M. and 6 P.M.
FINDING APY AND APR We use the general compound interest formula
1
⎛⎞
=+
⎜⎟
⎝⎠
nt
r
AP
n
and Example 4 of Section 2.1 of Calculus Concepts to illustrate the use of the
general compound interest formula.
To find the APR compounded monthly if an investment of
$10,000 is to grow to $1,000,000 in 40 years, substitute P =
10,000, n = 12, and t = 40 in the general formula. Enter the
resulting expression of
12(40)
10,000 1
12
⎛⎞
+
⎜⎟
⎝⎠
r
in Y1.
Access the solver by pressing MATH 0. We want to solve the
equation
1, 000, 000 =
12(40)
10,000 1
12
⎛⎞
+
⎜⎟
⎝⎠
r
for r. Subtract 1,000,000
from each side to obtain the equation 0 =
12(40)
10,000 1
12
⎛⎞
+
⎜⎟
⎝⎠
r
-
1,000,000. Since we have the expression
12(40)
10,000 1
12
⎛⎞
+
⎜⎟
⎝⎠
r
in
Y1, this is equivalent to 0 = Y1 – 1,000,000.
Press ENTER . With the cursor in the R location, enter a guess –
say 0.05.
Copyright © Houghton Mifflin Company. All rights reserved.
44
To Next Page
Loading...
Need help?
General
