Chapter 10
Beca
use the value of
P at the close points is more than its value at the critical point, w ≈ 0.492 whey
protein,
s ≈ 0.508 skim milk powder, and P ≈ 10.277% cooking loss is a minimum point.
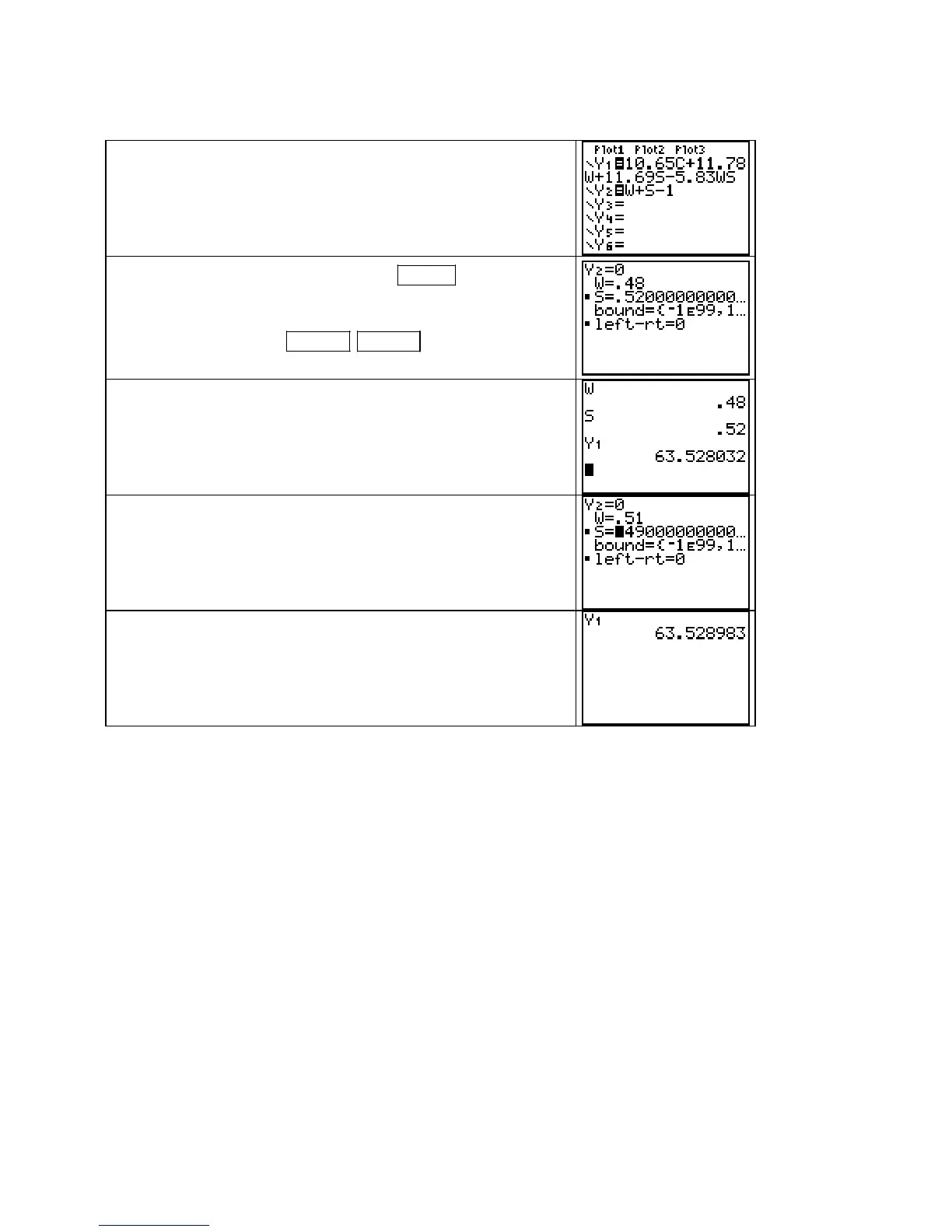
Enter
P(w, s) in Y1. Solve the constraint ( , ) 1gws
for 0 and
enter ( , ) 1 1
ws w s−= +−in Y2.
Access the EQUATION SOLVER with MATH 0 . Enter the
eqn: 0 = Y2. Press enter to see the screen at right. Choose a
value of
w that is less than 0.492, say 0.48. Store 0.48 in W. Go
to the S= line and press
ALPHA ENTER (SOLVE) . The
calculator finds the value of s on the constraint is
0.52s
.
Go to the home screen. If you like, you can check to see that the
calculator has stored the appropriate values for w and s. Use Y1
to evaluate P(0.48, 0.52). At this close point, the value of P is
more than the value of P at the critical point ( 63.527
).
Go back to the equation solver and choose a value of W that is
more than 0.492, say 0.51. Put your cursor on the S line and
solve for s. The calculator finds the value of s on the constraint
to be .
0.49s =
Go to the home screen and evaluate (0.51, 0.49) 63.529. P
Copyright © Houghton Mifflin Company. All rights reserved.
114
To Previous Page
Loading...
Need help?
General
