Chapter 10
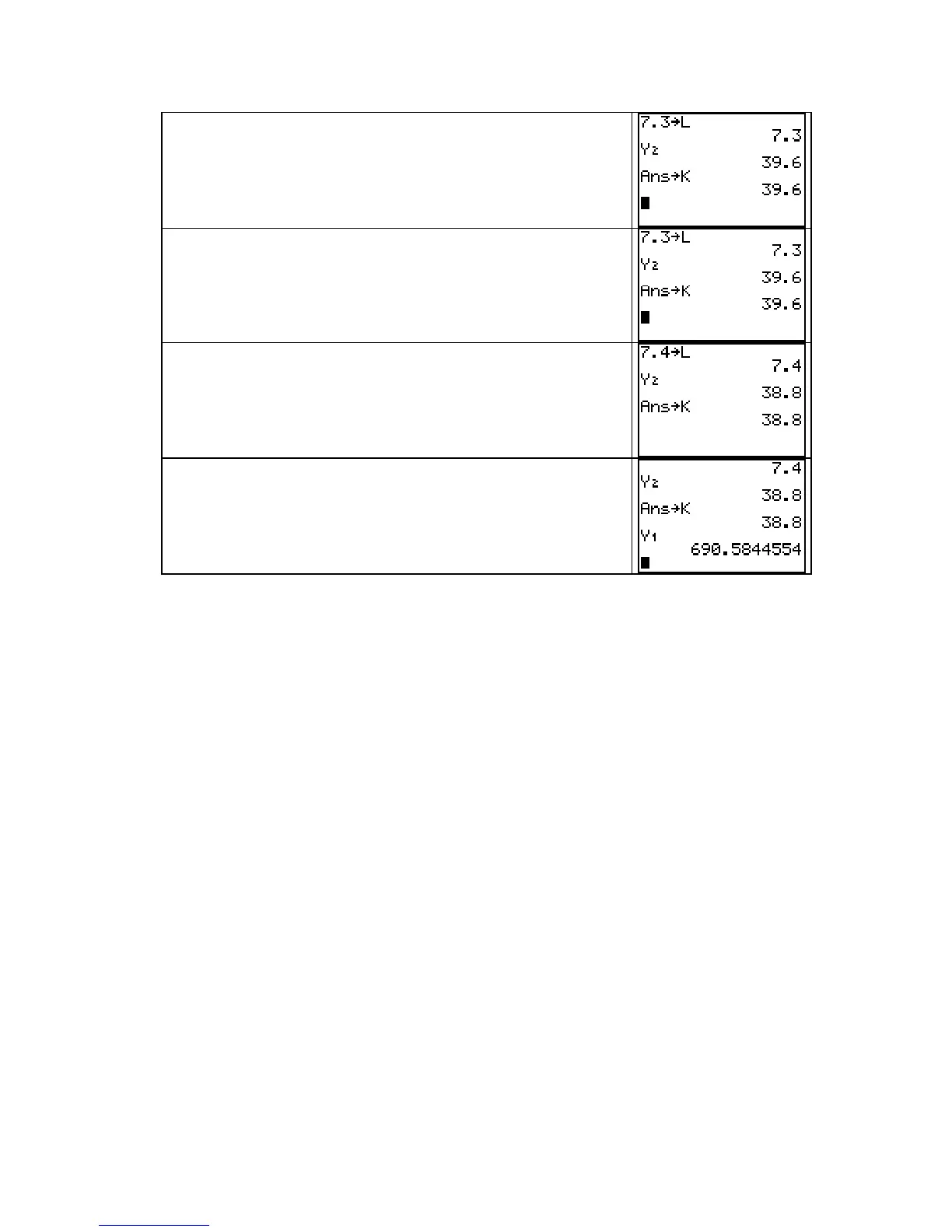
Choose a value of
L that is less than L = 7.35, say 7.3. Find the
value of
K so that 8L + K = 98. Remember that K = Y2, so store
7.3 in
L and call up Y2. Store this value in K.
Then find the value of f at L = 7.3, K = 39.6.
At this close point, the value of f is less than the value of f at the
critical point (690.6084798…).
Choose another value of L, this time one that is more than L =
7.35, say 7.4. Find the value of
K so that 8L + K = 98 by storing
7.4 in
L and calling up Y2. Store this value in K.
Then find the value of f at L = 7.4, K = 38.8.
At this close point, the value of f is less than the value of f at the
critical point (690.6084798…). Thus, (7.35, 39.2, 690) is a
maximum point for mattress production.
NOTE: Because the constraint is the equation in Y2, when we call up Y2 in the procedure
above we are finding points
on the constraint g. If you have the constraint in a different
location, you need to use the constraint location in the
Y= list to find the value of K.
Copyright © Houghton Mifflin Company. All rights reserved.
112
To Next Page
Loading...
Need help?
General
