Chapter 2
These values are those in the fifth row of the above table
− the values for k = 0.001. From this
point forward, we use k = 0.001 and therefore do not specify k when evaluating
nDeriv(. Will
the slope of this secant line always do a good job of approximating the slope of the tangent
line when k = 0.001? Yes, it generally does, as long as the instantaneous rate of change exists
at the input value at which you evaluate
nDeriv(. When the instantaneous rate of change does
not exist,
nDeriv( should not be used to approximate something that does not have a value.
You will benefit from reading again pages 46 and 47 of this
Guide, which illustrate several cases when the calculator’s slope
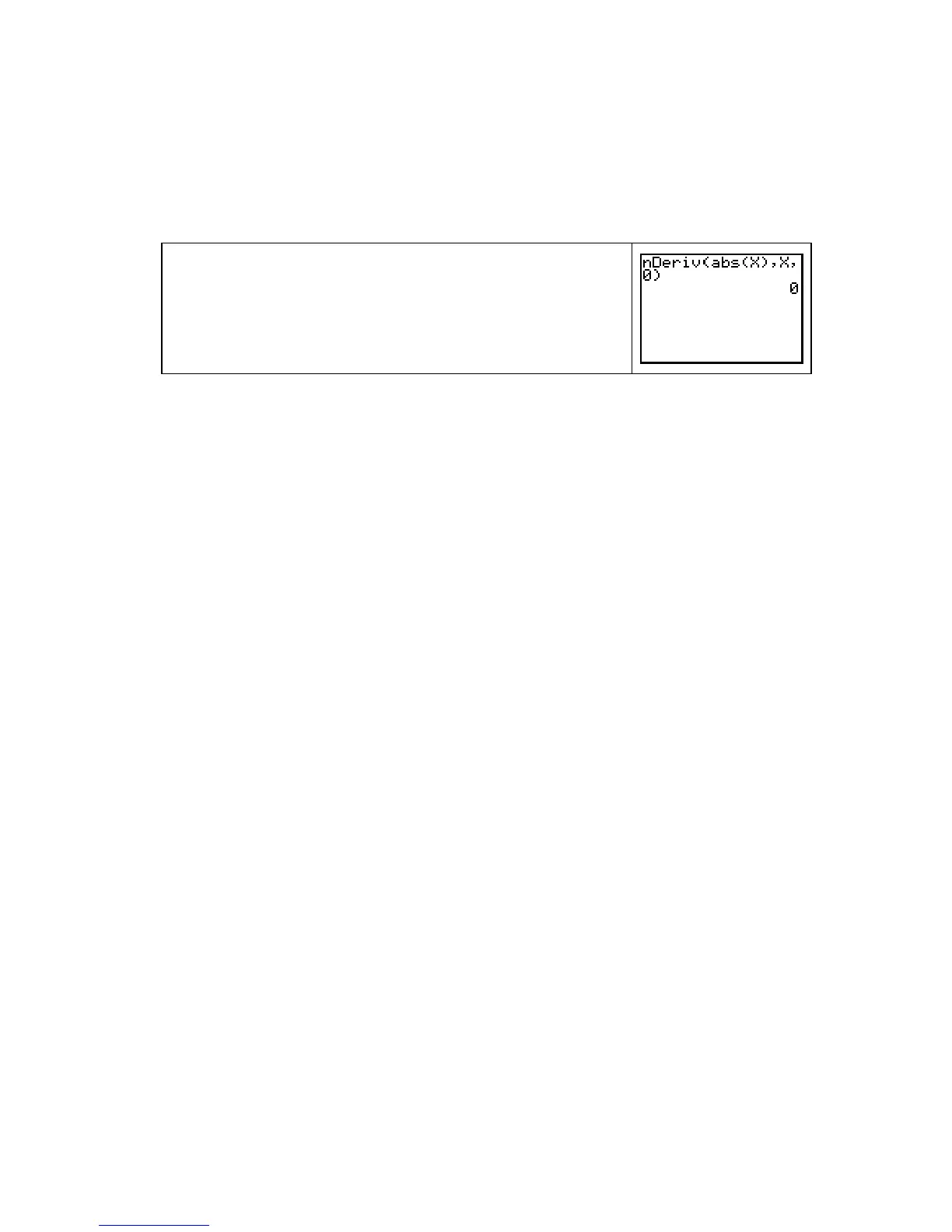
function gives a value for the slope when it does not exist. For
instance, the instantaneous rate of change of f(x) = |x| does not
exist at x = 0 because the graph of f has a sharp point there.
However, the calculator’s slope function does exist at that point.
Copyright © Houghton Mifflin Company. All rights reserved.
54

 Loading...
Loading...

















