Square-root raised cosine filters
Many communication systems use distributed filtering, that is, filtering is
performed partially in the transmitter, to limit bandwidth, and partially in the
receiver, to limit interference. To achieve the overall desired frequency response
each filter’s transfer function is based on the square root of the desired response.
For these systems matched square-root raised cosine filters are used in the
transmitter and the receiver sections of the system to achieve optimum signal to
noise ratio. This implies that you must select similar filter characteristics in the
analyzer (which simulates the receiver) to the filter characteristics of the
transmitter. The standard NADC and JDC demodulation types offered in this
analyzer provide this type of filter.
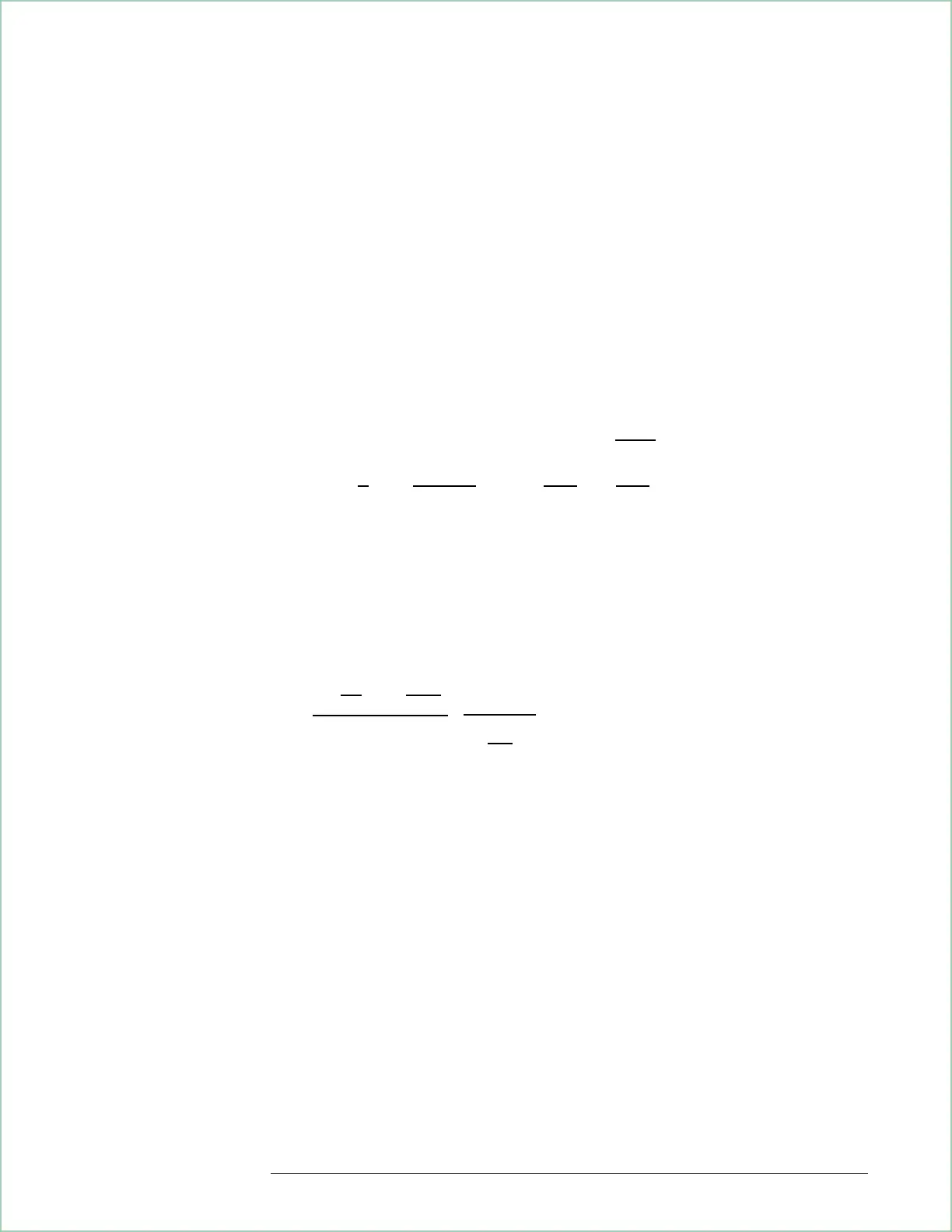
The equation for the square-root raised cosine (root Nyquist) filter follows:
++
H(f) =
1 when 0 ≤ f ≤
(1−α)
2T
√
1
2
1−sin
π(2fT−1)
2 α
when
1−α
2T
≤ f ≤
1+α
2T
0 when otherwise
Raised cosine filters
Raised cosine filters are used in systems which perform all the filtering in the
transmitter. This is typical of some mobile communication systems.
The equation for the raised cosine (Nyquist) filter follows:
++
h(t)=
sin
π t
T
cos
απt
T
π t
.
1
1−
2 α
T
t
2
Digital Demodulation Concepts (Opt. AYA)
22 - 17
++ Where T is the symbol interval
To Next Page
Loading...
Need help?
General
