Math setups FFT windows
FFT windows
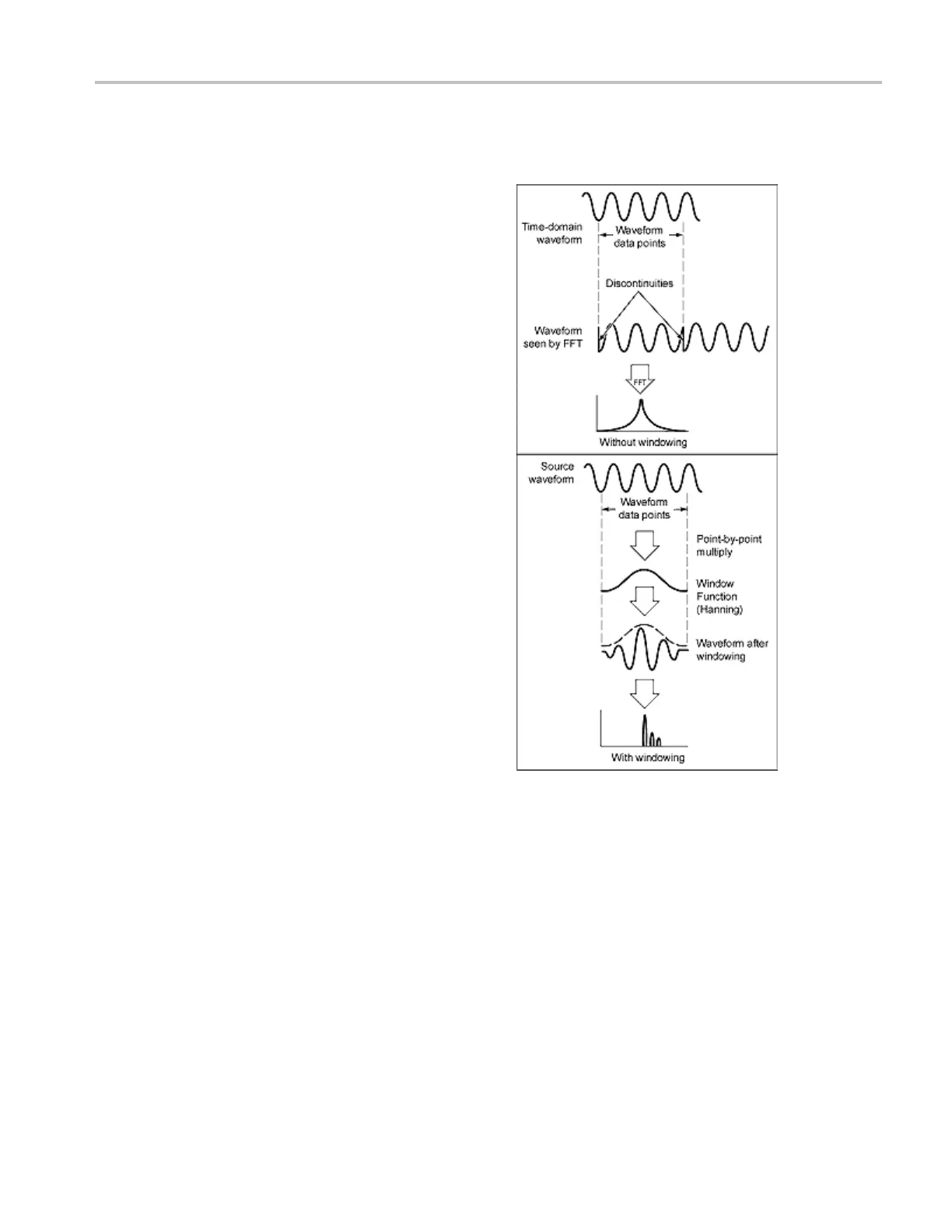
The FFT process assumes that the part of the waveform
record used fo
r FFT analysis represents a repeating
waveform that starts and ends at or near the same voltage
of a cycle. In other words, it is an integer number of cycles.
When a wavefo
rm starts and ends at the same amplitude,
there are no artificial discontinuities in the signal shape, and
both the frequency and amplitude information is accurate.
A nonintegr
al number of cycles in the waveform record
causes the waveform start and end points to be at different
amplitudes. The transitions between the start and end
points caus
e discontinuities in the waveform that introduce
high-frequency transients. These transients add false
frequency information to the frequency domain record.
Applying a
window function to the waveform record changes
the waveform so that the start and stop values are close to
each other, reducing the discontinuities. This results in an
FFT measu
rement that more accurately reflects the actual
signal frequency components. The shape of the window
determines how well it resolves frequency or magnitude
informat
ion.
xxx
DSA/DPO70000D, MSO/DPO/DSA70000C, DPO7000C, and MSO/DPO5000 Series 253
To Next Page
Loading...
Need help?
General
