Hioki PW8001-01 User Manual

213
Specications of Equations
Wiring
Item
1P2W 1P3W 3P3W2M 3V3A 3P3W3M 3P4W
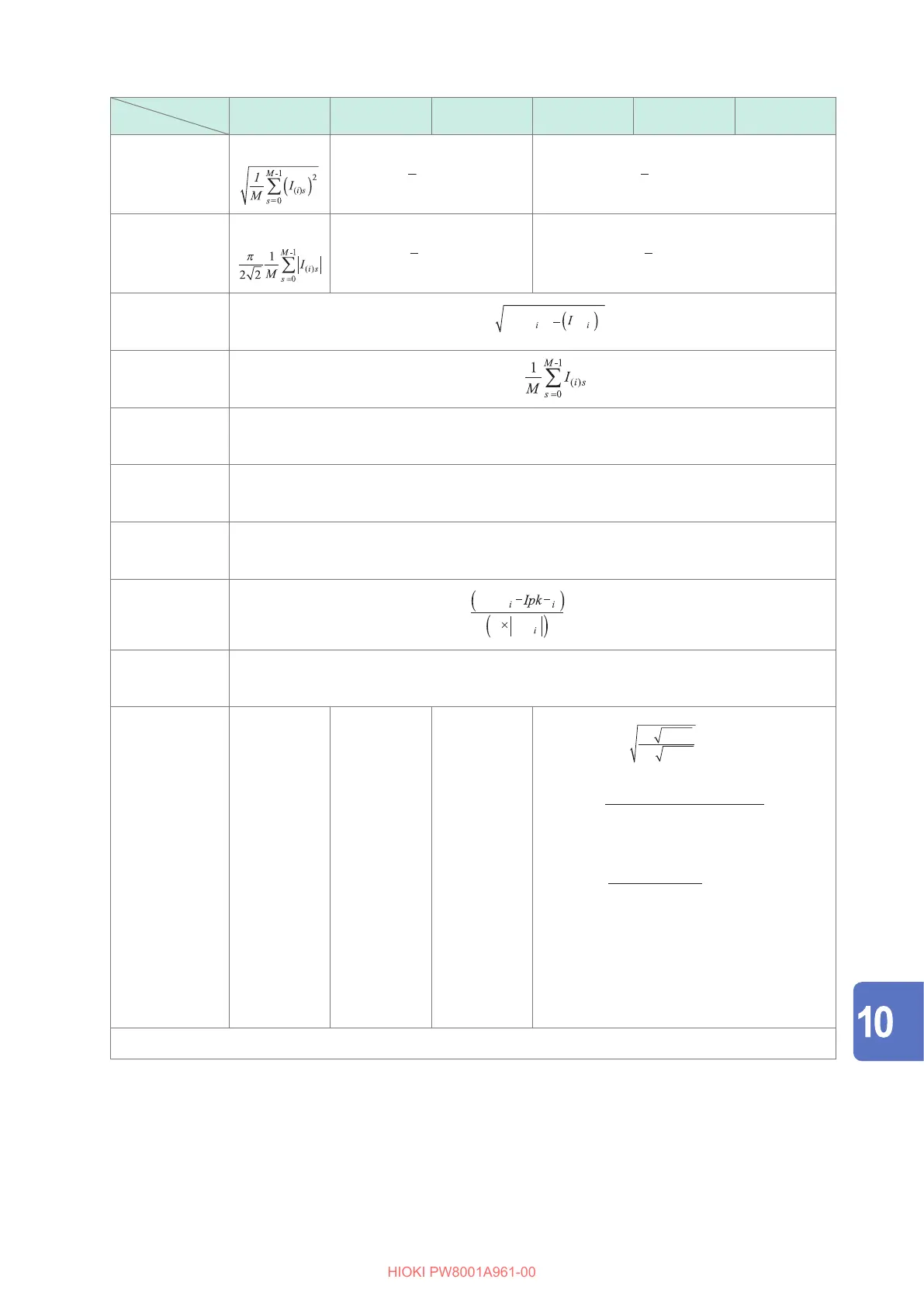
Current RMS
value
Irms
(i)
=
Irms
(i)(i+1)
=
1
2
(Irms
(i)
+ Irms
(i+1)
) Irms
(i)(i+1)(i+2)
=
1
3
(Irms
(i)
+ Irms
(i+1)
+ Irms
(i+2)
)
RMS equivalent
of average
rectied current
value
Imn
(i)
=
Imn
(i)(i+1)
=
1
2
(Imn
(i)
+ Imn
(i+1)
) Imn
(i)(i+1)(i+2)
=
1
3
(Imn
(i)
+ Imn
(i+1)
+ Imn
(i+2)
)
Current AC
component
Iac
(i)
=
()
Irms dc
2
)
2
()(
Current simple
average
Idc
(i)
=
Current
fundamental
wave component
Harmonic current
I
1(i)
in harmonic equation
Current peak
Ipk+
(i)
= I
(i)s
maximum of
M
data points
Ipk−
(i)
= I
(i)s
maximum of
M
data points
Total current
harmonic
distortion
Ithd
(i)
in harmonic equation
Current ripple
factor
Ipk+
Idc
×100
2
)( )(
)( )( )( )(
Current phase
angle
θI
1(i)
in harmonic equation
Current
unbalance rate
Iunb
(i)(i+1)(i+2)
=
1− 3−6
1+ 3−6
×100
β
β
β
=
++
)(
I I I
(i)(i+1)
2
(i+1)(i+2)
2
(i+2)(i)
2
2
++
I I I
(i)(i+1)
4
(i+1)(i+2)
4
(i+2)(i)
4
Example: When Ch. 1 to Ch. 3 are used
β
=
++
++
()
III
III
12
4
23
4
31
4
12
2
23
2
31
2
2
•
I
12
,
I
23
, and
I
31
are fundamental wave voltage
RMS values (line voltage), which are obtained
from harmonic calculation results.
• In 3P3W3M and 3P4W wiring mode, current is
converted to line current before calculation.
(i)
: measurement channel,
M
: number samples between sync timings,
s
: sample point number
Specications
HIOKI PW8001A961-00

Hioki PW8001-01 Specifications

General

Brand	Hioki
Model	PW8001-01
Category	Measuring Instruments
Language	English