STMicroelectronics STM32WL5 Series User Manual

Public key accelerator (PKA) RM0453
708/1450 RM0453 Rev 5
These values allow the recipient to compute the exponentiation m = A
d
(mod pq) more
efficiently as follows:
• m
1
= A
dP
mod p
• m
2
= A
dQ
mod p
• h = q
inv
(m
1
- m
2
) mod p, with m
1
> m
2
• m = m
2
+ hq
Operation instructions for computing CRT exponentiation A
d
mod pq are summarized in
Table 158.
24.4.14 Point on elliptic curve Fp check
This operation consists in checking whether a given point P (x, y) satisfies or not the curves
over prime fields equation y
2
= (x
3
+ ax + b) mod p, where a and b are elements of the
curve.
Operation instructions for point on elliptic curve Fp check are summarized in Table 159.
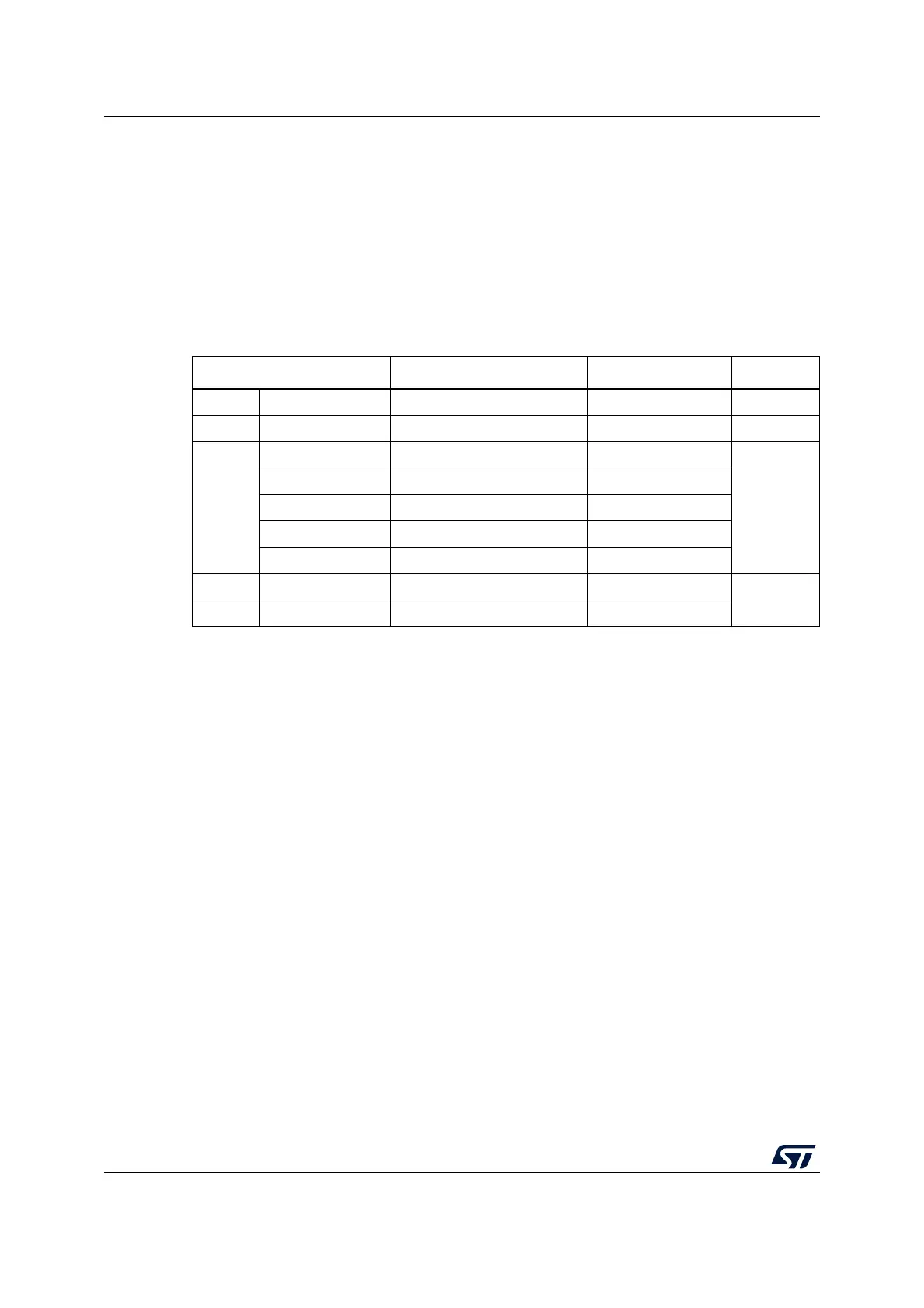
Table 158. CRT exponentiation
Parameters with direction Value (Note) Storage Size
IN MODE 0x07 PKA_CR 6 bits
IN Operand length (in bits, not null) RAM@0x404 32 bits
IN
Operand d
P
(0 ≤ d
P
< 2
M/2
)RAM@0x65C
ROS/2
Operand d
Q
(0 ≤ d
Q
< 2
M/2
)RAM@0xBD0
Operand q
inv
(0 ≤ q
inv
< 2
M/2
) RAM@0x7EC
Prime p
(1)
(0 ≤ p < 2
M/2
)RAM@0x97C
Prime q
(1)
(0 ≤ q< 2
M/2
)RAM@0xD5C
IN Operand A (0 ≤ A< 2
M/2
) RAM@0xEEC
ROS
OUT Result: A
d
mod pq (0 ≤ result < pq)RAM@0x724
1. Must be different from 2.

STMicroelectronics STM32WL5 Series Specifications

General

Brand	STMicroelectronics
Model	STM32WL5 Series
Category	Microcontrollers
Language	English